We thought we were hot stuff...
In 1985, Goldman Sachs plucked me from Northwestern University's Kellogg School of Management to be part of a team of elite specialists it was building for a curious assignment: to develop the best ways of making money in the markets, while slashing risk to an absolute minimum.
This group included MBAs from the best business schools... PhDs in mathematics, computer science, economics, and physics... and even a rocket scientist from NASA. We had access to everything we could possibly need to accomplish this goal.
During that time, a colleague of mine came incredibly close to reaching our goal. His name was Dr. Fischer Black... a Harvard math specialist who'd once worked for a U.S. government think tank.
Fischer was shy... and kept to himself. I don't recall him attending a single one of our office parties.
But he developed a unique algorithm... We used it to make hundreds of thousands of dollars every single day. And it forms the basis of the options trading we do in my newsletter, Retirement Trader.
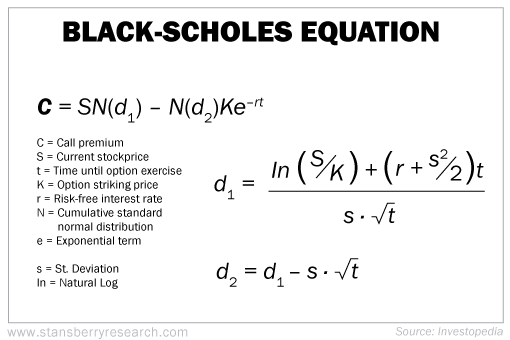
If you're interested, here's what his Nobel Prize-winning Black-Scholes equation looks like...
Elementary, right?
Options often seem like a foreign concept for many folks who are just starting to trade them. But an internalized understanding of options is invaluable.
That's why we're going to break this algorithm into several basic principles. Today, we'll start with the strike price.
In Retirement Trader, we sell put and call options. For the purposes of this discussion, we'll focusing on buying call options. It's a bit easier and more intuitive.
Focus on the Strike Price
The strike price is the agreed-upon price at which the owner of the option can buy the underlying stock. This is different from the option price, which is what the buyer pays to own the option.
Let's look at someone who buys a call option with a strike price of $100 per share. If the stock rises to, say, $200 a share before the option expires, the option holder would make a profit (depending on the initial purchase price, of course). He could buy the stock for $100 per share, then turn right around and sell it for $200 a share. So if he paid $4 per option contract, he would make at least $96.
That's straightforward. But it demonstrates some interesting properties of options...
For instance, consider the option price. The $100-strike option will be worth very little if the stock is trading at $10. The likelihood of the option paying off is clearly lower if shares need to go from $10 to $100.
Conversely... what if the stock were currently trading at $120 a share? That option would be worth at least $20 a share with absolute certainty. That's because the owner of that option can exercise it immediately, buy the shares for $100, and sell them in the market for $120. Thus, the "option" has to be at least worth $20.
This measure is the "intrinsic value." (Any other value that comes from the remaining time is the "extrinsic" or "time" value.)
Now think about this...
Consider when the stock rises from $10 a share to $11 a share. Would an option buyer consider that a big change in the likelihood that an option with a $100 strike would pay off?
I wouldn't... That option is so far away from the strike price that a $1 rise in the share price doesn't make it any more likely the stock is going to surpass $100 a share.
However, if the stock is sitting at $98 share, a $1 rise to $99 a share would make a meaningful difference. The likelihood of a payout has changed significantly.
If that doesn't jump out to you, let's make the example more extreme. If the stock moves $10, say from $5 to $15, that doesn't make much difference. However, if it goes from $88 to $98, that clearly does. It's much more likely to hit the strike price.
The point is that not all changes in the stock price are created equal.
Congratulations – you now understand the concept of "delta."
Delta measures how the option price changes in response to a given change in stock price.
In our example, the change from $10 a share to $11 a share would have a delta of nearly zero. The change from $98 a share to $99 a share would be closer to 0.5, but on its way to 1.
Here's a real-world example:
| Microsoft (MSFT) August Calls | |
| Strike | Delta |
| $420 | 0.77 |
| $440 | 0.62 |
| $445 | 0.59 |
| $470 | 0.35 |
Your broker's platform should list the delta for each option. You can also see delta numbers for free at www.nasdaq.com.
When we pulled these numbers, Microsoft traded for $447 a share. You can see, the $420 calls are likely to pay off, so they have a higher delta. For every $1 that Microsoft shares move, we'd expect this option to move $0.77.
Meanwhile, you've still got a long way to go before the $470 call really has a good chance of paying off. If Microsoft shares rise $1, this option will only rise $0.35.
Understanding delta is the first step to truly understanding options and how much you stand to gain or lose with stock-price changes. Knowing this puts you far ahead of the rest of investors.
Last week, I released a video where I show a pro golfer exactly how to use my Retirement Trader strategy to collect thousands of dollars in income... instantly.
If you've ever been curious about how to use options in your own portfolio, click here to watch my demonstration before it goes offline tonight.
What We're Reading...
- The secret to safely making huge income streams all comes down to gambling.
- Something different: The Celtics win a record 18th NBA championship.
Here's to our health, wealth, and a great retirement,
Dr. David Eifrig and the Health & Wealth Bulletin Research Team
June 20, 2024